Have you heard about the ‘conic section’ ?. No!. Let me tell you, a conic section is a section of a curve that is obtained when a cone intersects with a plane surface. A hyperbola is a type of conic section which can be regarded as a curve formed by the intersection of two curves or a double curve with a plane surface. This intersection may not take place in the center of the hyperbola. It can also be defined as a curve that is smooth and lies in a figure which is plane. A hyperbola is made up of various parts such as focus, center, the minor, axis and major axis, vertices, and so on. There are various types of hyperbolas but all of them possess the same characteristics such as: made up of two curves, a focus, and vertex. In this article, we may cover some basic aspects of a hyperbola such as parts of a hyperbola, applications of a hyperbola, and do a detailed analysis about it.
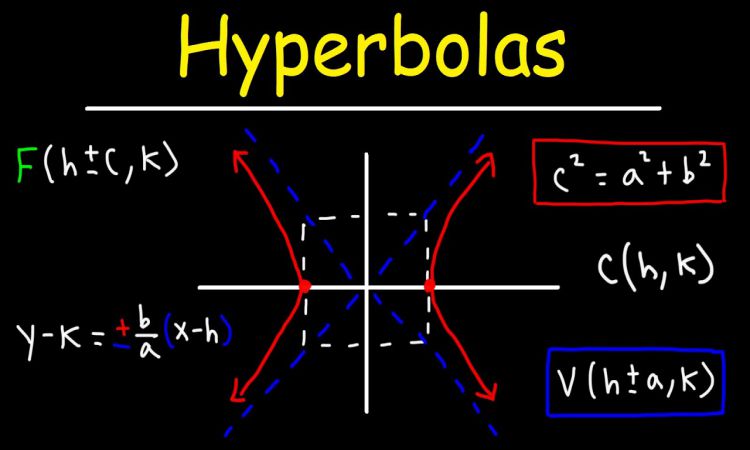
Some Parts of a Hyperbola
As mentioned above, a hyperbola is a type of conic section which can be regarded as a curve formed by the intersection of two curves or a double curve with a plane surface.
The following points analyze the parts of a hyperbola in a detailed manner:
- The two fixed points inside the curve of a hyperbola are regarded as the foci of a hyperbola. The singular term of foci is ‘focus’.
- A point that joints the foci ( two ) can be defined as the center of the hyperbola.
- The length of hyperbola measuring 2a units is known as the major axis and the length of hyperbola measuring 2b units is known as the minor axis of hyperbola.
- A point where the hyperbola meets with the axis ( major and minor ) can be defined as the vertices of the hyperbola.
- A line that passes from or through both the foci ( two ) and center of a hyperbola is known as the transverse axis. The line that passes from the two foci and is perpendicular in nature with respect to the transverse axis is defined as the conjugate axis of hyperbola.
- The distance from the focus to the midpoint which is known as the center of a hyperbola is measured in ratios and thus known as the eccentricity of the hyperbola.
Some Applications of Hyperbola in Real life
Some of the applications of a hyperbola in real life are discussed below:
- The shape of the hyperbola helps in the design of a bridge. For example, a bridge in Japan was made with the help of a hyperbola.
- Hyperbola is considered as the basis of solving the problem of trilateration (GPS-related problems ).
- Do you know what a sundial is ?. A sundial is a horological device that is used to measure time. Hyperbola can be seen in those sundials. More often than not, they are also used in flashlights.
Master Math from Online Math Classes
There are several topics in mathematics that are difficult to understand. If you do not understand a topic or a segment, you may be in a situation where you feel anxiety. Do not worry, Cuemath is there to help you. It is a leading personalized platform found across 20 countries. Cuemath provides you with highly qualified and experienced teachers who will help you to grasp difficult concepts in a better way. The teachers also provide you with various math puzzles and worksheets which will help you study math in a fun way. Therefore, if you want to study online math classes from Cuemath, you should visit the website of Cuemath and understand math, the Cuemath way. Book a free session now.
